24 Sep Material level Hierarchical VVUQ Strategy
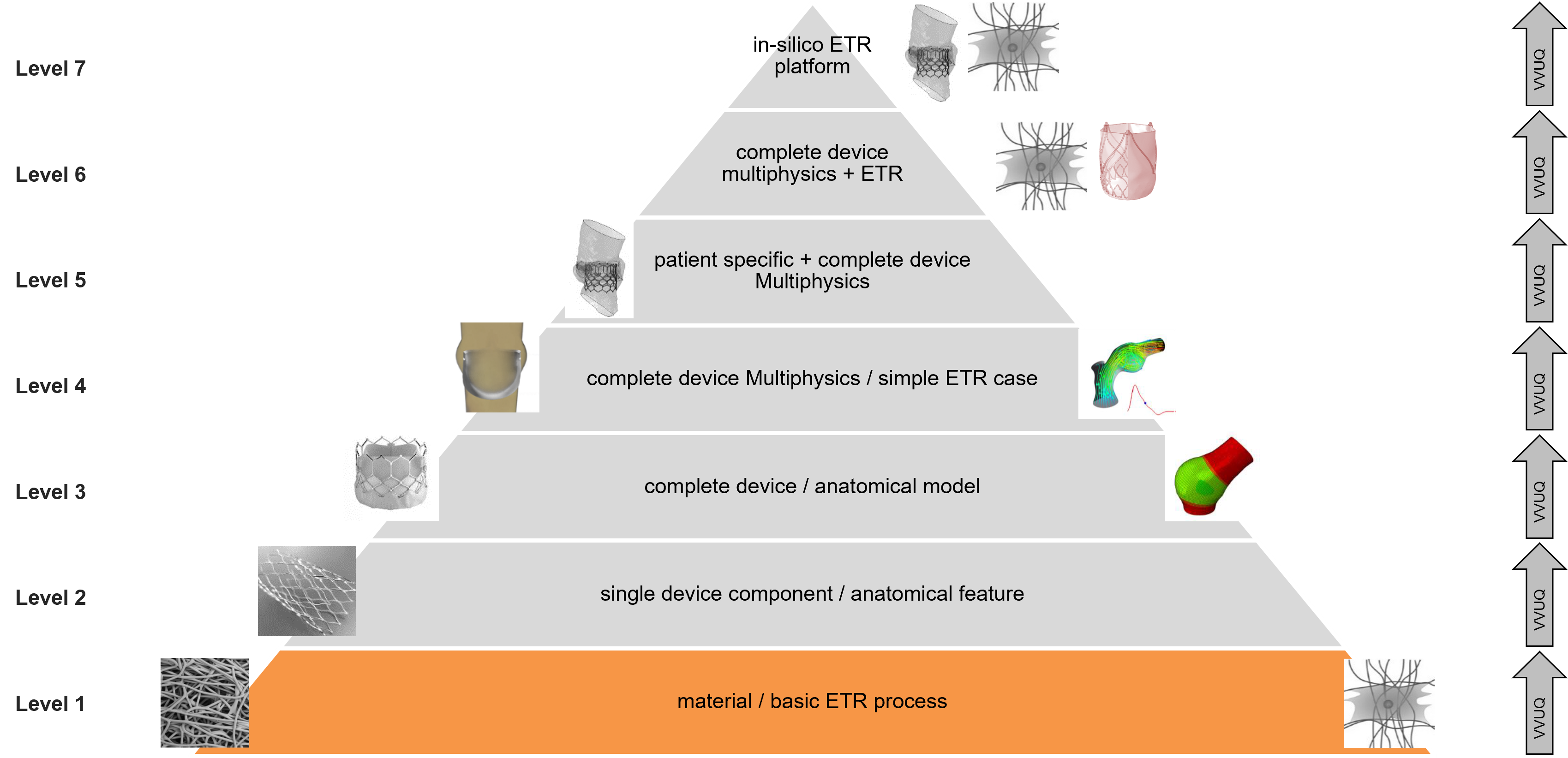
Material Level in Hierarchical VVUQ Strategy for Biodegradable Pulmonary Heart Valve Development
Welcome to the second post in our series on the Hierarchical VVUQ Strategy for the development of biodegradable pulmonary heart valves as part of the EU-funded SimInSitu project. Today, we delve into the first level of the VVUQ pyramid: the Material Level. This level is crucial as it forms the foundation for developing and calibrating the material models needed for the pulmonary valve (PV) model.
Read the first blog post: Hierarchical VVUQ Strategy
Read the third blog post: Single device level Hierarchical VVUQ Strategy
Read the fourth blog post: Complete Device LevelHierarchical VVUQ Strategy
Material Characterization
At the material model level, we needed to develop and calibrate two material models: one for the leaflet and another for the conduit scaffold materials. Despite their similarities in general composition and overall mechanical behavior, these materials were tailored to meet specific performance and safety requirements.
To characterize these scaffold materials, we employed uniaxial and biaxial tensile cyclic loading tests, as well as uniaxial stress relaxation tests. Material coupons were manufactured from sheets of cylindrical scaffold types, with five samples per material and test mode, oriented in both circumferential and axial directions. The resulting stress-strain (and time) data confirmed the hyperelastic, anisotropic, and viscoelastic nature of the materials, along with the well-pronounced Mullin’s effect and plastic set point.
The Representative (filtered and averaged) experimental stress-strain curves for the scaffold material showing the characteristic material properties are shown below.

Additionally, as part of a Round Robin study within the SimInSitu consortium, these uniaxial and biaxial tests were conducted across several laboratories to assess the uncertainty arising from experimental test execution.
Constitutive Modelling
To model the hyperelastic anisotropic response of the scaffold material, we introduced a polyconvex function based on the invariants of second-order structural tensors. Inelastic effects, including stress softening and permanent deformation, were modeled using a pseudo-elastic function combined with a finite strain viscoelastic model. The developed scaffold material model was then successfully implemented in Abaqus through user-subroutines UMAT and VUMAT. The figure below shows a representative numerical stress-strain curve for a calibrated scaffold material in axial and circumferential direction.

Code and Calculation Verification
We verified our model’s numerical implementation by comparing the numerical solutions from Abaqus with analytical solutions for two test cases: a biaxial tensile test and a simple shear test. Additionally, we used Cook’s membrane problem for calculation verification, examining the convergence of the numerical solution with different element formulations during several mesh refinement steps. These verification activities were successfully completed.
Material Calibration
For parameter identification and uncertainty quantification, we employed a probabilistic approach using Bayesian inference. The results demonstrated good qualitative agreement between experimental data and theoretical predictions, even accounting for significant variability in the experimental results. The model approximated various inelastic effects well on a global scale, though local quantitative agreement depended on the chosen calibrated stretch regime. The Round Robin data were also considered in the calibration to incorporate uncertainties in the experimental setup.
Model Validation
To validate our model, we conducted an experimental parallel plate test using five samples for each of the three types of scaffold materials. Each tube-shaped sample measured either 6mm or 23mm in diameter. We also developed a computational model to simulate the parallel plate test in Abaqus as shown below. Using the computational model’s output, we performed a comprehensive global sensitivity analysis and uncertainty quantification to identify influential parameters.
The validation assessment compared different sets of material parameters identified for small and large strain regimes. Although some shortcomings were identified, we achieved satisfactory validation results, particularly for the larger 23mm diameter samples.

Conclusion
The material level forms the bedrock of our hierarchical VVUQ strategy, providing a solid foundation for subsequent levels. In our next post, we will explore the single device component level, where we focus on modeling, verification, and validation of individual device components. Stay tuned!


